8.4 Mid-point of a line | Analytical geometry

8.4 Mid-point of a line (EMA6H)

Finding the mid-point of a line

On graph paper, accurately plot the points \(P\left(2;1\right)\) and \(Q\left(-2;2\right)\) and draw the line \(PQ\).

Fold the piece of paper so that point \(P\) is exactly on top of point \(Q\).
Where the folded line intersects with line \(PQ\), label point \(S\)
Count the blocks and find the exact position of \(S\).
Write down the coordinates of \(S\).

To calculate the coordinates of the mid-point \(M\left(x;y\right)\) of any line between the points \(A\left({x}_{1};{y}_{1}\right)\) and \(B\left({x}_{2};{y}_{2}\right)\), we use the following formulae:

\begin{align*} x & = \frac{{x}_{1} + {x}_{2}}{2} \\ y & = \frac{{y}_{1} + {y}_{2}}{2} \end{align*}

From this we obtain the mid-point of a line:

Mid-point \(M\left(x;y\right) = \left(\dfrac{{x}_{1}+{x}_{2}}{2} \; ; \; \dfrac{{y}_{1}+{y}_{2}}{2}\right)\)

This video shows some examples of finding the mid-point of a line.

Video: 2GDF

Worked example 10: Calculating the mid-point

Calculate the coordinates of the mid-point \(F\left(x;y\right)\) of the line between point \(E\left(2;1\right)\) and point \(G\left(-2;-2\right)\).

Draw a sketch

From the sketch, we can estimate that \(F\) will lie on the \(y\)-axis, with a negative \(y\)-coordinate.

Assign values to \(\left({x}_{1};{y}_{1}\right)\) and \(\left({x}_{2};{y}_{2}\right)\)

\[{x}_{1} = -2 \quad {y}_{1} = -2 \quad {x}_{1} = 2 \quad {y}_{2}=1\]

Write down the mid-point formula

\[F\left(x;y\right) = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right)\]

Substitute values into the mid-point formula

\begin{align*} x & = \frac{{x}_{1} + {x}_{2}}{2} \\ & = \frac{-2 + 2}{2} \\ & = 0 \\ y & = \frac{{y}_{1} + {y}_{2}}{2} \\ & = \frac{-2 + 1}{2} \\ & = -\frac{1}{2} \end{align*}

Write the answer

The mid-point is at \(F\left(0;-\frac{1}{2}\right)\).

Looking at the sketch we see that this is what we expect for the coordinates of \(F\).

For worked example 10 (calculating the mid-point) learners can check their answer using the distance formula.

Using the distance formula, we can confirm that the distances from the mid-point to each end point are equal:

\begin{align*} PS & = \sqrt{{\left({x}_{1} - {x}_{2}\right)}^{2} + {\left({y}_{1} - {y}_{2}\right)}^{2}} \\ & = \sqrt{{\left(0 - 2\right)}^{2} + {\left(-\text{0,5} - 1\right)}^{2}} \\ & = \sqrt{{\left(-2\right)}^{2} + {\left(-\text{1,5}\right)}^{2}} \\ & = \sqrt{4 + \text{2,25}} \\ & = \sqrt{\text{6,25}} \end{align*}

and

\begin{align*} QS & = \sqrt{{\left({x}_{1}-{x}_{2}\right)}^{2}+{\left({y}_{1}-{y}_{2}\right)}^{2}} \\ & = \sqrt{{\left(0-\left(-2\right)\right)}^{2} + {\left(-\text{0,5}-\left(-2\right)\right)}^{2}} \\ & = \sqrt{{\left(0 + 2\right)}^{2}{+\left(-\text{0,5} + 2\right)}^{2}} \\ & = \sqrt{{\left(2\right)}^{2}{+\left(-\text{1,5}\right)}^{2}} \\ & = \sqrt{4 + \text{2,25}} \\ & = \sqrt{\text{6,25}} \end{align*}

As expected, \(PS=QS\), therefore \(F\) is the mid-point.

Worked example 11: Calculating the mid-point

Find the mid-point of line \(AB\), given \(A\left(6;2\right)\) and \(B\left(-5;-1\right)\).

Draw a sketch

From the sketch, we can estimate that \(M\) will lie in quadrant I, with positive \(x\)- and \(y\)-coordinates.

Assign values to \(\left({x}_{1};{y}_{1}\right)\) and \(\left({x}_{2};{y}_{2}\right)\)

Let the mid-point be \(M\left(x;y\right)\)

\[{x}_{1} = 6 \quad {y}_{1} = 2 \quad {x}_{2} = -5 \quad {y}_{2} = -1\]

Write down the mid-point formula

\[M\left(x;y\right) = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right)\]

Substitute values and simplify

\[M\left(x;y\right) = \left(\frac{6 - 5}{2};\frac{2 - 1}{2}\right) = \left(\frac{1}{2};\frac{1}{2}\right)\]

Write the final answer

\(M\left(\frac{1}{2};\frac{1}{2}\right)\) is the mid-point of line \(AB\).

We expected \(M\) to have a positive \(x\)- and \(y\)-coordinate and this is indeed what we have found by calculation.

temp text

Worked example 12: Using the mid-point formula

The line joining \(C\left(-2;4\right)\) and \(D\left(x;y\right)\) has the mid-point \(M\left(1;-3\right)\). Find point \(D\).

Draw a sketch

From the sketch, we can estimate that \(D\) will lie in Quadrant IV, with a positive \(x\)- and negative \(y\)-coordinate.

Assign values to \(\left({x}_{1};{y}_{1}\right)\) and \(\left({x}_{2};{y}_{2}\right)\)

Let the coordinates of \(C\) be \(\left({x}_{1};{y}_{1}\right)\) and the coordinates of \(D\) be \(\left({x}_{2};{y}_{2}\right)\).

\[{x}_{1} = -2 \quad {y}_{1} = 4 \quad {x}_{2} = x \quad {y}_{2} = y\]

Write down the mid-point formula

\[M\left(x;y\right) = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right)\]

Substitute values and solve for \({x}_{2}\) and \({y}_{2}\)

\[\begin{array}{rlcrl} 1 & = \dfrac{-2 + {x}_{2}}{2} & \qquad\qquad & -3& = \dfrac{4 + {y}_{2}}{2} \\ 1\times 2& = -2 + {x}_{2} & \qquad\qquad & -3 \times 2 & = 4 + {y}_{2} \\ 2 & = -2 + {x}_{2} & \qquad\qquad & -6 & = 4 + {y}_{2} \\ {x}_{2} & = 2 + 2 & \qquad\qquad & {y}_{2} & = -6 - 4 \\ {x}_{2} & =4 & \qquad\qquad & {y}_{2} & = -10 \end{array}\]

Write the final answer

The coordinates of point \(D\) are \(\left(4;-10\right)\).

temp text

Worked example 13: Using the mid-point formula

Points \(E\left(-1;0\right)\), \(F\left(0;3\right)\), \(G\left(8;11\right)\) and \(H\left(x;y\right)\) are points on the Cartesian plane. Find \(H\left(x;y\right)\) if \(EFGH\) is a parallelogram.

Draw a sketch

Method: the diagonals of a parallelogram bisect each other, therefore the mid-point of \(EG\) will be the same as the mid-point of \(FH\). We must first find the mid-point of \(EG\). We can then use it to determine the coordinates of point \(H\).

Assign values to \(\left({x}_{1};{y}_{1}\right)\) and \(\left({x}_{2};{y}_{2}\right)\)

Let the mid-point of \(EG\) be \(M\left(x;y\right)\)

\[{x}_{1} = -1 \quad {y}_{1} = 0 \quad {x}_{2} = 8 \quad {y}_{2} = 11\]

Write down the mid-point formula

\[M\left(x;y\right) = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right)\]

Substitute values calculate the coordinates of \(M\)

\[M\left(x;y\right) = \left(\frac{-1 + 8}{2};\frac{0 + 11}{2}\right) = \left(\frac{7}{2};\frac{11}{2}\right)\]

Use the coordinates of \(M\) to determine \(H\)

\(M\) is also the mid-point of \(FH\) so we use \(M\left(\dfrac{7}{2};\dfrac{11}{2}\right)\) and \(F\left(0;3\right)\) to solve for \(H\left(x;y\right)\).

Substitute values and solve for \(x\) and \(y\)

\[\begin{array}{rlcrl} \dfrac{7}{2} & = \dfrac{0 + x}{2} & \qquad \qquad & \dfrac{11}{2} & = \dfrac{3+y}{2} \\ 7 & = x + 0 & \qquad \qquad & 11 & = 3 + y \\ x & = 7 & \qquad \qquad & y & = 8 \end{array}\]

Write the final answer

The coordinates of \(H\) are \(\left(7;8\right)\).

Textbook Exercise 8.5

You are given the following diagram:

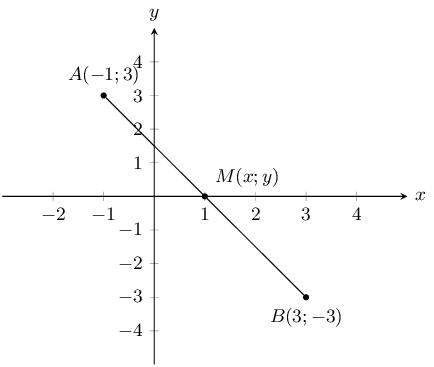
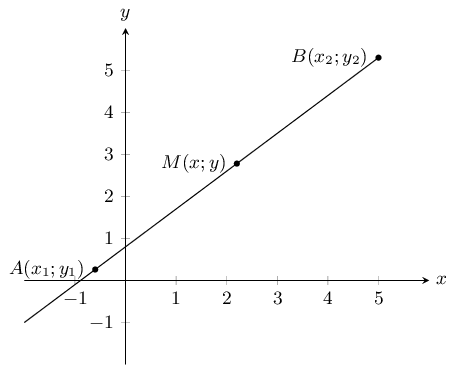
Calculate the coordinates of the mid-point (\(M\)) between point \(A (-\text{1};\text{3})\) and point \(B (\text{3};-\text{3})\).

Let the coordinates of \(A\) be \(\left({x}_{1};{y}_{1}\right)\) and the coordinates of \(B\) be \(\left({x}_{2};{y}_{2}\right)\).

\[{x}_{1} = -1 \quad {y}_{1} = 3 \quad {x}_{2} = 3 \quad {y}_{2} = -3\]

Substitute values into the mid-point formula:

\begin{align*} M\left(x;y\right) & = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right) \\ x & = \frac{{x}_{1} + {x}_{2}}{2} \\ & = \frac{-1 + 3}{2} \\ & = 1 \\ y & = \frac{{y}_{1} + {y}_{2}}{2} \\ & = \frac{3 + (-3)}{2} \\ & = 0 \end{align*}

The mid-point is at \(M\left(1;0\right)\).

You are given the following diagram:

Calculate the coordinates of the mid-point (\(M\)) between point \(A (-\text{2};\text{1})\) and point \(B (\text{1};-\text{3,5})\).

Let the coordinates of \(A\) be \(\left({x}_{1};{y}_{1}\right)\) and the coordinates of \(B\) be \(\left({x}_{2};{y}_{2}\right)\).

\[{x}_{1} = -2 \quad {y}_{1} = 1 \quad {x}_{2} = 1 \quad {y}_{2} = -\text{3,5}\]

Substitute values into the mid-point formula:

\begin{align*} M\left(x;y\right) & = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right) \\ x & = \frac{{x}_{1} + {x}_{2}}{2} \\ & = \frac{-2 + 1}{2} \\ & = -\text{0,5} \\ y & = \frac{{y}_{1} + {y}_{2}}{2} \\ & = \frac{1 + (-\text{3,5})}{2} \\ & = -\text{1,25} \end{align*}

The mid-point is at \(M\left(-\text{0,5};-\text{1,25}\right)\).

\(A(2;5)\), \(B(-4;7)\)

\begin{align*} M_{AB} &= \left(\frac{x_1 + x_2}{2};\frac{y_1 + y_2}{2}\right) \\ &= \left(\frac{2 - 4}{2};\frac{5 + 7}{2}\right) \\ &= \left(\frac{-2}{2};\frac{12}{2}\right) \\ &= (-1;6) \end{align*}

\(C(5;9)\), \(D(23;55)\)

\begin{align*} M_{CD} &= \left(\frac{x_1 + x_2}{2};\frac{y_1 + y_2}{2}\right) \\ &= \left(\frac{5 + 23}{2};\frac{9 + 55}{2}\right) \\ &= \left(\frac{28}{2};\frac{64}{2}\right) \\ &= (14;32) \end{align*}

\(E(x + 2;y - 1)\), \(F(x - 5;y - 4)\)

\begin{align*} M_{EF} &= \left(\frac{x_1 + x_2}{2};\frac{y_1 + y_2}{2}\right) \\ &= \left(\frac{x + 2 + x - 5}{2};\frac{y - 1 + y - 4}{2}\right) \\ &= \left(\frac{2x - 3}{2};\frac{2y - 5}{2}\right) \end{align*}

The mid-point \(M\) of \(PQ\) is \((3;9)\). Find \(P\) if \(Q\) is \((-2;5)\).

The mid-point formula is:

\[M\left(x;y\right) = \left(\frac{{x}_{1} + {x}_{2}}{2};\frac{{y}_{1} + {y}_{2}}{2}\right)\]

Substituting values and solving for \({x}_{2}\) and \({y}_{2}\) gives:

\[\begin{array}{rlcrl} 3 & = \dfrac{-2 + {x}_{2}}{2} & \qquad\qquad & 9 & = \dfrac{5 + {y}_{2}}{2} \\ 6 & = -2 + {x}_{2} & \qquad\qquad & 18 & = 5 + {y}_{2} \\ {x}_{2} & = 6 + 2 & \qquad\qquad & {y}_{2} & = 18 - 5 \\ {x}_{2} & = 8 & \qquad\qquad & {y}_{2} & = 13 \end{array}\]

The coordinates of point \(P\) are \(\left(8;13\right)\).

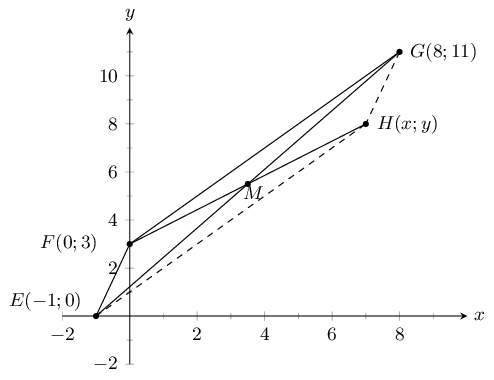
\(PQRS\) is a parallelogram with the points \(P(5;3)\), \(Q(2;1)\) and \(R(7;-3)\). Find point \(S\).

Draw a sketch:

The diagonals of a parallelogram bisect each other, therefore the mid-point of \(QR\) will be the same as the mid-point of \(PS\). We must first find the mid-point of \(QR\). We can then use it to determine the coordinates of point \(H\).

\begin{align*} M_{QR} &= \left(\frac{x_1 + x_2}{2};\frac{y_1 + y_2}{2}\right)\\ &= \left(\frac{2 + 7}{2};\frac{1 - 3}{2}\right) \\ &= \left(\frac{9}{2};\frac{-2}{2}\right) \\ &= \left(\frac{9}{2};-1\right) \end{align*}

Use mid-point \(M\) to find the coordinates of \(S\):

\begin{align*} M_{QR} &= (\frac{x_1 + x_2}{2};\frac{y_1 + y_2}{2}) \\ \left(\frac{9}{2};-1\right) &= \left(\frac{x + 5}{2};\frac{y + 3}{2}\right) \end{align*}

Solve for \(x\):

\begin{align*} \frac{9}{2} &= \frac{x + 5}{2} \\ 9 &= x + 5 \\ x &= 4 \end{align*}

Solve for \(y\):

\begin{align*} -1 &= \frac{y + 3}{2} \\ -2 &= y + 3 \\ y &= -5 \end{align*}

Therefore \(S(4;-5)\).

8.3 Gradient of a line

Table of Contents

8.5 Chapter summary